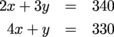The unknown quantities are Anna's speed and Bob's speed. We can tell this from the first and last parts of the problem. The problem says "Whenever Anna drives, she goes one speed." If we weren't going to be asked to find Anna's speed, the problem could have just told us how fast she drives, and if the problem wasn't dead set on being sneaky, it would have. Also, towards the end of the problem, it asks "How fast does Anna drive?" That's almost screaming out "Anna's speed is an unknown quantity that we want to find!" Once again, a problem is screaming something out when it could have just as easily gotten the information across by speaking in a calm, controlled manner. Let's say x is Anna's speed, and y is Bob's speed. Now we need the two pieces of actual information that are given to us. In this problem, the first piece of information is what happened on the first day. If Anna drove for 2 hours and Bob drove for 3, then the two traveled 2x + 3y miles. Our first equation is therefore 2x + 3y = 340. The second piece of information is what happened on the second day: Anna drove 4 hours and Bob drove 1, and they went 450 miles, so 4x + y = 330. If x is Anna's speed and y is Bob's speed, then the problem is described by the system of equations 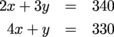
|