Solving an equation that contains rational expressions is similar to solving any old equation that has fractions in it. In fact, if the variable only occurs in numerators, we actually are solving a equation with fractions in it. Our apologies to those of you who were psyched for something completely new and challenging. You'll need to get your kicks elsewhere.
Remember that, with fractions, the denominator tells us the size of the pieces, and the numerator tells us how many pieces we have. In order for two fractions with the same denominator to be equal, the numerators must also be the same.
Two pieces of size eight and three pieces of size eight will never be the same amount. For two pans of brownies to have the same amount of brownie goodness in them when the pieces are cut the same size, each pan must also have the same number of brownies. PS, in case you now absolutely must make a pan of brownies, we like this recipe.
Sample Problem
Solve the equation  .
.
Since the denominators are equal, the numerators must be equal, too. Ever since the Variable Rights Act, anyway. The only value of x that will work is 3.
Sample Problem
Solve the equation  .
.
There are two ways to do this.
Way 1: Eliminate the denominators. If you've got a bottle of Denominator-Off, that would work best. If not, have no fear. We can still make this happen.
If we multiply each side of the equation by 5, we find  .
.
If we then multiply each side of the equation by 6, we get 18 = 5x, which means  .
.
Way 2: Put the fractions over a common denominator so that we can compare the numerators, in the same way that it's easier to compare the size of two hot dogs when they're sitting on the same plate in front of you. If you've got one dog in your hand and the other at the opposite end of a football field, it'll be harder to tell the size difference. By the way, it seems to us you're misusing that football field.
The LCD of the two fractions is 5 × 6 = 30, so we can rewrite the left side of the equation like this:

And we can rewrite the right side of the equation as:

Now we solve the equation  , which is equivalent to the original equation. Since the denominators are now the same, we need the numerators to be the same. We're in a "same" kind of mood right now.
, which is equivalent to the original equation. Since the denominators are now the same, we need the numerators to be the same. We're in a "same" kind of mood right now.
We must have 18 = 5x, so divide both sides by 5 to finish up:

Both ways of solving the equation led us to the exact same answer. When asked to solve an equation involving rational expressions, you can use either method, whichever one floats your canoe:
- Eliminate the denominators, or
- Write the expression on each side of the equation as a fraction (where the fractions have the same denominator).
Now it's time to take off the gloves. They were scrunching our fingers anyway. We'll allow the variables into the denominators this time as well. Sorry, denominators. Your club is exclusive no more. You need to keep up with the times.
When there are variables in denominators, the first thing to do is figure out which values of the variables make the denominators equal to zero. These values can't be solutions to the equation; we can't even evaluate both sides of the equation at these values. Unfortunately, we may need to factor to find the "bad" values of the variable. Then we'll give them an after-school detention and send a note home to their parents.
Sample Problem
The value x = 2 can't possibly be a solution to the equation  , since the left-hand side of the equation isn't even defined at x = 2. It's true; we checked dictionary.com and it wasn't there.
, since the left-hand side of the equation isn't even defined at x = 2. It's true; we checked dictionary.com and it wasn't there.
Similarly, we can factor the denominator on the right-hand side to see which values won't work over there:
x2 + 2x + 1 = (x + 1)(x + 1)
We see that x = -1 can't be a solution because the right-hand side of the equation isn't defined at that point.
After finding the "bad" values (naughty, naughty values), we can solve an equation with rational expressions using either of the two ways mentioned above. If you're having trouble deciding, flip a coin. If you're having trouble deciding which coin to use, roll a die. If you're still having trouble, we won't be able to help you. Those are the only ways we know to decide things.
Sample Problem
Solve the equation  .
.
First we check for "bad" values: x = 1 isn't allowed in this equation, since that would make more than one of our denominators zero. We already don't like it when one denominator equals zero. Can you imagine our wrath if two of them had that problem? You wouldn't like us when we're angry.
Now on to solving. We know you're raring to go. While we could add the expressions on the left-hand side of the equation and then put both sides of the equation over a common denominator, that sounds like too much work. For an easier way out, we'll stick with Way 1. To eliminate denominators, we multiply both sides of the equation by (1 – x). This gives us:
x – 1(1 – x) = 1
And that simplifies to x – 1 + x = 1, or 2x = 2.
This looks like x = 1 should be a solution to the equation. Alas, we found that x = 1 was a "bad" value. It can't be a solution after all, so the poor equation has no solutions. Maybe we can take up a collection for it. Sadface.
The value x = 1 in the example above was an extraneous solution, or a value that pretends to be a solution but can't actually be one because it's a "bad" value. Unlike a regular old "bad" value that's up to no good, an extraneous solution pretends to be your friend at the same time, which is even worse. Can you say "betrayal"?
Be careful: Check for extraneous solutions. Look for "bad" values before you solve a rational equation. After you solve the rational equation, only keep those solutions that aren't "bad" values. Keep them in a safe place, where the "bad" values can't get to them and turn them to the Dark Side.
Exercise 1
Solve the following equation or state that the equation has no solutions, remembering to check for extraneous solutions:  .
.
Exercise 2
Solve the following equation or state that the equation has no solutions, remembering to check for extraneous solutions:  .
.
Exercise 3
Solve the following equation or state that the equation has no solutions, remembering to check for extraneous solutions:  .
.
Exercise 5
Solve the following equation or state that the equation has no solutions, remembering to check for extraneous solutions:  .
.
 .
.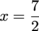 (the solution
(the solution  aren't "bad" values. We should also make sure that they're not seeing each other, so we can be assured they're not having a "bad" romance.
aren't "bad" values. We should also make sure that they're not seeing each other, so we can be assured they're not having a "bad" romance. and the right-hand side of the original equation by
and the right-hand side of the original equation by  . This gives us an equivalent equation:
. This gives us an equivalent equation:
 .
. , which means that -7 and 9 are "bad" values that can't possibly be solutions.
, which means that -7 and 9 are "bad" values that can't possibly be solutions.
