Definite Integrals Exercises

Answer

To find the length of a sub-interval, we take the length of the original interval (4) and divide by the number of sub-intervals we want to chop it into (8).

• On each sub-interval, we go to the right endpoint of that sub-interval and go up until we hit the function. The y-value of the function there is the height of the rectangle on that sub-interval.

• We need to find the area of each rectangle.

Sub-interval [0, 0.5]:

The right endpoint of this sub-interval is 0.5. The height of this rectangle is

f(0.5) = (0.5)² + 1 = 1.25

so the area is

(height) ⋅ (width) = 1.25 ⋅ (0.5) = 0.625

Sub-interval [0.5, 1]:

The right endpoint of this sub-interval is 1. The height of this rectangle is

f(1) = 1² + 1 = 2

so the area is

(height) ⋅ (width) = 2 ⋅ (0.5) = 1

Sub-interval [1, 1.5]:

The right endpoint of this interval is 1.5. The height of this rectangle is

f(1.5) = (1.5)² + 1 = 3.25

so the area is

(height) ⋅ (width) = 3.25 ⋅ (0.5) = 1.625

Sub-interval [1.5, 2]:

The right endpoint of this interval is 2. The height of this rectangle is

f(2) = 2² + 1 = 5

so the area is

(height) ⋅ (width) = 5 ⋅ (0.5) = 2.5

Sub-interval [2, 2.5]:

The right endpoint of this interval is 2.5. The height of this rectangle is

f(2.5) = (2.5)² + 1 = 7.25

so the area is

(height) ⋅ (width) = 7.25 ⋅ (0.5) = 3.625.

Sub-interval [2.5, 3]:

The right endpoint of this interval is 3. The height of this rectangle is

f(3) = 3² + 1 = 10

so the area is

(height) ⋅ (width) = 10 ⋅ (0.5) = 5.

Sub-interval [3, 3.5]:

The right endpoint of this interval is 3.5. The height of this rectangle is

f(3.5) = (3.5)² + 1 = 13.25

so the area is

(height) ⋅ (width) = 1 ⋅ (0.5) = 6.625

Sub-interval [3.5, 4]:

The right endpoint of this sub-interval is 4. The height of this rectangle is

f (4) = (4)² + 1 = 17

so the area is

(height) ⋅ (width) = 17 ⋅ (0.5) = 8.5.

Adding the areas of all 8 rectangles, we get

0.625 + 1 + 1.625 + 2.5 + 3.625 + 5 + 6.625 + 8.5 = 29.5.

• We can see that the rectangles are covering R and then some.

This means the area covered by the rectangles is greater than the area of R, so we have an overestimate.

Answer

• If we use two sub-intervals, the length of a sub-interval is .

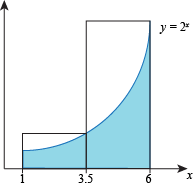
The subintervals are then [1, 3.5] and [3.5, 6].

On the first sub-interval the height of the rectangle is

f(3.5) = 2^3.5

and the width of the rectangle is 2.5 (the length of the sub-interval), so the area of the first rectangle is

2^3.5(2.5) ≈ 28.28

On the second sub-interval the height of the rectangle is

f(6) = 2⁶ = 64

and the width is 2.5, so the area of this second rectangle is

64(2.5) = 160.

Adding the areas of the rectangles together, we estimate that the area of S is approximately

28.28 + 160 = 188.28.

• Dividing the interval [1, 6] into 5 sub-intervals gives us sub-intervals of length 1, so each rectangle will have width 1.

Sub-interval [1, 2]:

The height of the rectangle is

f(2) = 2² = 4

so the area of the rectangle is

height ⋅ width = 4(1) = 4.

Sub-interval [2, 3]:

The height of the rectangle is

f(3) = 2³ = 8

so the area of the rectangle is

height ⋅ width = 8(1) = 8.

Sub-interval [3, 4]:

The height of the rectangle is

f(4) = 2⁴ = 16

so the area of the rectangle is

height ⋅ width = 16(1) = 16.

Sub-interval [4, 5]:

The height of the rectangle is

f (5) = 2⁵ = 32

so the area of the rectangle is

height ⋅ width = 32(1) = 32.

Sub-interval [5, 6]: The height of the rectangle is

f(6) = 2⁶ = 64

so the area of the rectangle is

height ⋅ width = 64(1) = 64.

Adding the areas of all these rectangles together, we estimate that the area of S is

4 + 8 + 16 + 32 + 64 = 124.

The approximations found in are both overestimates, as we can see the rectangles cover all of S and then some.

Answer

Dividing the interval [1, 4] into 3 sub-intervals of equal length gives us sub-intervals of length 1.

Sub-interval [1, 2]:

The height of this rectangle is

so the area of the rectangle is

Sub-interval [2, 3]:

The height of this rectangle is

so the area of the rectangle is

Sub-interval [3, 4]:

The height of this rectangle is

so the area of the rectangle is

Adding the areas of these rectangles, we estimate that the area of W is

.

Dividing the interval [1, 4] into 6 sub-intervals of equal length gives us sub-intervals of length .

Sub-interval [1, 1.5]:

The height of this rectangle is

so the area is

Sub-interval [1.5, 2]:

The height of this rectangle is

so the area is

Sub-interval [2, 2.5]:

The height of this rectangle is

so the area is

Sub-interval [2.5, 3]:

The height of this rectangle is

so the area is

Sub-interval [3, 3.5]:

The height of this rectangle is

so the area is

Sub-interval [3.5, 4]:

The height of this rectangle is

so the area is

.

Adding the areas of all these rectangles, we estimate that the area of W is

• The approximations are both underestimates, since the rectangles do not cover the entire area of W.

Answer

If we use only one sub-interval, that means we're not breaking up the original interval up at all. We will have one rectangle of width 6 and height

f (8) = 20.

The area of this rectangle is

height ⋅ width = 20(6) = 120.

Since this is the only rectangle we're using, we estimate that the area between the graph of f and the x-axis is 120.

To use three sub-intervals we need to break the original interval [2, 8] into sub-intervals of length 2.

On sub-interval [2, 4] the height of the rectangle is f(4) = 5 so the area of this rectangle is

height ⋅ width = 5(2) = 10.

On sub-interval [4, 6] the height of the rectangle is f(6) = 12 so the area of this rectangle is

height ⋅ width = 12(2) = 24.

On sub-interval [6, 8] the height of the rectangle is f(8) = 20 so the area of this rectangle is

height ⋅ width = 20(2) = 40.

Adding the areas of all the rectangles, we estimate that the area between the graph of f and the x-axis is

10 + 24 + 40 = 74.

• The estimates in are over-estimates.

Since the function f is increasing, it must look something like this:

The rectangles must be covering extra area that isn't between the graph of f and the x axis:

Answer

• With 3 sub-intervals we split the original interval [-1, 2] into sub-intervals of length 1, so each rectangle will have width 1.

On [-1, 0] the rectangle has height g(0) = 20 and area 20(1) = 20.

On [0, 1] the rectangle has height g(1) = 18 and area 18(1) = 18.

On [1, 2] the rectangle has height g(2) = 3 and area 3(1) = 3.

Adding the areas of these rectangles, we estimate that the area between the graph of g(x) and the x-axis on [-1, 2] is

20 + 18 + 3 = 41.

With 2 sub-intervals we split the original interval [-1, 2] into sub-intervals of length 1.5.On [-1, 0.5] the rectangle has height g (0.5) = 19 and width 1.5, so its area is

19(1.5) = 28.5.

On [0.5, 2] the rectangle has height g(2) = 3 and width 1.5, so its area is

3(1.5) = 4.5.

Adding the areas of these rectangles, we estimate that the area between the graph of g (x) and the x-axis on [-1, 2] is

28.5 + 4.5 = 33

The estimates are both underestimates. The function g (x) and the rectangles from first estimate would look something like this:

The rectangles don't cover the entire area between g and the x-axis on [-1, 2], so we get an underestimate of that area.

Answer

We divide the interval [-20, 20] into 4 sub-intervals of size 10. Each rectangle has width 10.

On sub-interval [-20, -10] the rectangle has height f(-10) = 5 (we read this off from the graph). The area of the rectangle is

height ⋅ width = 5(10) = 50.

On sub-interval [-10, 0] the rectangle has height f(0) = 10 (we read this off from the graph). The area of the rectangle is

height ⋅ width = 10(10) = 100.

On sub-interval [0, 10] the rectangle has height f(10) = 0. The area of the rectangle is 0.

On sub-interval [10, 20] the rectangle has height f(20) = 20. The area of the rectangle is

height ⋅ width = 20(10) = 200.

Adding together the areas of the rectangles, we estimate that the area of W is

50 + 100 + 0 + 200 = 350.

Answer

We divide the interval [-4, 0] into two sub-intervals of size 2, so each rectangle has width 2.

On sub-interval [-4, -2] the rectangle has height g(-2) = 4, so the area of this rectangle is 4(2) = 8.

On sub-interval [-2, 0] the rectangle has height g(0) = 6, so the area of this rectangle is

6(2) = 12.

We estimate that the area of Z is

8 + 12 = 20.

Now we'll divide the interval [-4, 0] into four sub-intervals of size 1, so each rectangle has width 1.

On sub-interval [-4, -3] the height of the rectangle is g(-3) = 2 so the area of the rectangle is 2(1) = 2.

On sub-interval [-3, -2] the height of the rectangle is g(-2) = 4 so the area of the rectangle is 4.

On sub-interval [-2, -1] the height of the rectangle is g(-1) = 6 so the area of the rectangle is 6.

On sub-interval [-1, 0] the height of the rectangle is g(0) = 6 so the area of the rectangle is 6.

Adding the areas of these rectangles, we estimate that the area of Z is

2 + 4 + 6 + 6 = 18.

• In both cases the rectangles cover Z and then some:

When we add the areas of the rectangles, we're getting more area than just the area of Z, so we get an overestimate.

Answer

Dividing the interval [-6, -3] into 6 sub-intervals produces sub-intervals of length -.5. We need the following values of f:

We add them up, and multiply by the width of a sub-interval:

[f (-5.5) + f (-5) + f (-4.5) + f (-4) + f (-3.5) + f (-3)](width) =

[6.25 + 4 + 2.25 + 1 + 0.25 + 0](.5) = 6.875

Answer

Dividing the interval [0, 4] into 8 sub-intervals produces sub-intervals of length 0.5.

We add these values of f and multiply by the width of a sub-interval:

[f (0.5) + f (1) + f (1.5) + f ( 2 )+ f (2.5) + f (3)+ f (3.5)+ f(4)](width)

= [8.75 + 9 + 8.75 + 8 + 6.75 + 5 + 2.75 + 0](0.5)

= 24.5

Answer

Breaking the interval [0, 12] into 3 sub-intervals gives us 3 sub-intervals of size 4. We need the following values of g, which we find by looking them up in the table:

We add these values and multiply by the width of a sub-interval:

Answer

Breaking the interval [-9, 9] into 9 sub-intervals gives us sub-intervals of length 2. We can look in the table to find the values of h at

x = -7, -5, -3, -1, 1, 3, 5, 7, 9.

We add these values and multiply by the width of a sub-interval:

[h(-7) + h(-5) + h(-3) + h(-1) + h(1) + h(3) + h(5) + h(7) + h(9)](width)

= [2 + 3 + 4 + 3 + 2 + 1 + 0 + 2 + 5](2)

= 44.

Answer

Breaking the interval [0, 30] into 3 sub-intervals gives us sub-intervals of length 10. From looking at the graph we can see that

We add these up and multiply by the length of a sub-interval:

Answer

From the table we can see that the sub-intervals we need to use are

[-10, -7], [-7, -6], [-6, -2], and [-2, 1].

These have lengths 3, 1, 4, and 3 respectively.

On the sub-interval [-10, -7] the rectangle has height f(-7) = 10 and width 3, so its area is 30.

On the sub-interval [-7, -6] the rectangle has height f(-6) = 7 and width 1, so its area is 7.

On the sub-interval [-6, -2] the rectangle has height f(-2) = 2 and width 4, so its area is 8.

On the sub-interval [-2, 1] the rectangle has height f(-1) = 1 and width 3, so its area is 3.

We estimate that the area between the graph of f and the x-axis on [-10, 1] is

30 + 7 + 8 + 3 = 48.