Think you’ve got your head wrapped around The Fundamental Theorem of Calculus? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. Let  For what values of x does F(x) = 0?
For what values of x does F(x) = 0?
 For what values of x does F(x) = 0?
For what values of x does F(x) = 0? x = 0
x = 2πk where k is any integer
x = πk where k is any integer
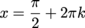 and
and 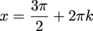 , where k is any integer.
, where k is any integer. Q. Let 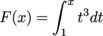 . For which values of x is F(x) positive?
. For which values of x is F(x) positive?
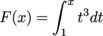 . For which values of x is F(x) positive?
. For which values of x is F(x) positive? x > 0
x > 1
|x| > 1
|x| < 1
Q. Define a function F(x) by

Which of the following best represents the value F(π)?
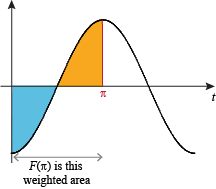
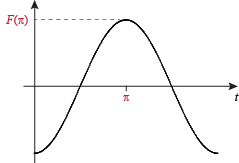

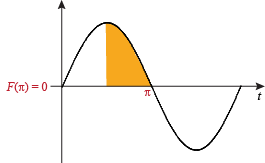
Q. Let f(x) be a continuous function. The second Fundamental Theorem of Calculus says that
the function 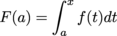 is an antiderivative of f(x).
is an antiderivative of f(x).
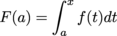 is an antiderivative of f(x).
is an antiderivative of f(x). the function  is an antiderivative of f(x).
is an antiderivative of f(x).
 is an antiderivative of f(x).
is an antiderivative of f(x). the function  is an antiderivative of f(x).
is an antiderivative of f(x).
 is an antiderivative of f(x).
is an antiderivative of f(x). the function 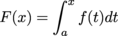 is an antiderivative of f(x).
is an antiderivative of f(x).
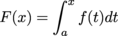 is an antiderivative of f(x).
is an antiderivative of f(x). Q. Which of the following is NOT an antiderivative of eex?

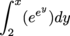
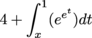

Q. Which of the following is an antiderivative of cos (x2) that equals 2 when x = π?
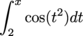
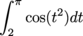


Q. Let 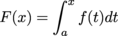 and
and  . Then
. Then 
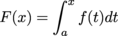 and
and  . Then
. Then 
0


f(b) – f(a)
Q. The equation 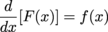 means
means
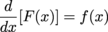 means
means F is an antiderivative of f.
f is an antiderivative of F.
F is the derivative of f.
f '(x) = F(x).
Q. 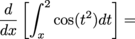
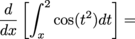
cos (x2)
-cos (x2)
cos(x2) – cos 4
2 – cos(x2)
Q. 

ex6
ex6 × 3x2
ex6 · 3x2
e(3x2)2