Word problems involving integrals usually fall into one of two general categories: alien related and non-alien related. The non-alien related ones are totally the worst. Here's how to figure them out.
1) The problem may state in words what you're supposed to find, in which case you have to translate those words into symbols.
Sample Problem
A snail travels with velocity
v(t) = 2 + 0.5sin t
feet per second, where t is given in seconds. To the nearest foot, how far does the snail travel from time  seconds to time t = 2π seconds?
seconds to time t = 2π seconds?
Answer.
The problem gives us a function for velocity and asks for distance travelled over a specified time interval. This means we need to use an integral.
To get the distance the snail travels, we integrate the velocity function over the specified time interval.

From  to t = 2π seconds, the snail travels approximately 9 feet.
to t = 2π seconds, the snail travels approximately 9 feet.
2) Alternately, the problem could provide the symbols and ask you to translate back into words.
Problems where you need to translate from English into integrals can get a little more complicated.
Instead of the words translating into an integral you need to evaluate, the words might translate into an equation you need to solve. Such an equation will probably involve an integral with letters in the integrand or in the limits of integration.
Example 1
A snail travels with velocity v(t) = 2 + 0.5sin t feet per second, where t is given in seconds. If the snail starts traveling at noon (t = 0), what does the expression represent in the context of this problem? |
Example 2
Water pressure p at a depth of h feet below the surface of the water is given by the formula p(h) = 62.4h measured in "pcf," or "pounds per cubic foot." Evaluate the integral
and explain what it means in terms of this problem. |
Example 3
Sonya can paint at a rate of v(t) = 150 – 4t square feet per hour, where t is the number of hours since she started painting. How long will it take Sonya to paint 568 square feet? |
Example 4
A pan of brownies has been prepared at room temperature, 70°F, and is put into an oven that has been pre-heated to 350°F. When the brownies have been in the oven for t minutes they heat up at a rate inversely proportional to (t + 1), with proportionality constant k. If the brownies are 250 degrees after being in the oven for 15 minutes, what is k? |
Exercise 1
Bob leaves for a trip at 3pm (time t = 0) and drives with velocity  miles per hour, where t is measured in hours. Find
miles per hour, where t is measured in hours. Find

and explain what it represents in the language of the problem.
Exercise 2
A faucet is turned on and water flows out at a rate of

gallons per minute, where t is the number of minutes since the faucet was turned on. To the nearest gallon, how much water flows out of the faucet during the first two minutes the faucet is turned on?
Exercise 3
Air pressure p at h meters above sea level is given by the formula
p(h) = 101,325(1 – 2.25577 × 10-5h)5.25588.
The quantity p(h) is measured in Pascals.
How much does air pressure change as one moves from 100 meters above sea level to 1000 meters above sea level?
Exercise 4
Fluffles the cat meows more loudly the higher she climbs in her favorite tree. Her volume changes at a rate of

decibels per foot, where h is Fluffles' height above ground measured in feet.
Find

and explain what it represents in the context of this problem.
Exercise 5
Sonya can paint at a rate of
v(t) = 150 – 4t
square feet per hour, where t is the number of hours since she started painting. Can Sonya paint the walls of a 12 foot by 12 foot office with an 8 foot high ceiling in 3 hours?
What about the walls of a 14 foot by 14 foot office with an 8 foot high ceiling?
Exercise 6
Gougem Airlines charges customers for checked bags based on weight. Checked bags cost $0.50 per pound up to 50 pounds, and $1 per pound for each additional pound. Let c(p) be the cost per pound for a checked bag, where p is the weight of the bag in pounds. Evaluate

and explain what it means in terms of Gougem Airlines.
Exercise 7
A ball is thrown at the ground from the top of a tall building. The speed of the ball in meters per second is
v(t) = 9.8t + v0,
where t denotes the number of seconds since the ball has been thrown and v0 is the initial speed of the ball (also in meters per second). If the ball travels 25 meters during the first 2 seconds after it is thrown, what was the initial speed of the ball?
Exercise 8
As one dives deeper, the water pressure p in pcf (pounds per cubic foot) at a depth of h feet below the surface of the water changes at a rate of
p'(h) = 62.4 pcf per foot.
If one starts at a depth of 15 feet below the surface of the water, how many feet deeper must one dive for the water pressure to increase by 800 pcf? Round your answer to the nearest foot.
Exercise 9
Shauna starts painting at noon. She can paint (140 – kt) square feet per hour, where t is the number of hours since she started painting and k is a constant accounting for the fact that Shauna slows down as she gets tired. If Shauna paints 100 square feet between 2pm and 3pm, what is k?
Exercise 10
Tom Sawyer is painting a fence at a rate of (200 – 4x) square feet per hour, where x is the number of hours since he started painting. If the fence is 800 square feet, how long will it take him to finish painting the fence? Round your answer to the nearest minute.
















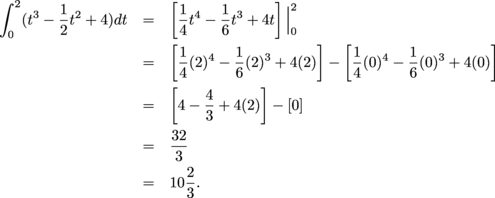



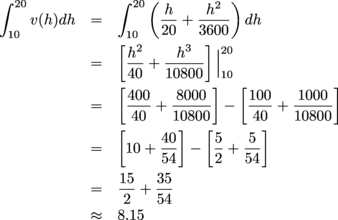










 square feet between 2pm and 3pm. Since the problem says she painted 100 square feet during this time interval, we must have
square feet between 2pm and 3pm. Since the problem says she painted 100 square feet during this time interval, we must have





