First, we should assign an angle to each side. A, B, and C will be the angles opposite the sides in increasing order. That means a = 10, b = 11, and c = 12. We'll find A first. a2 = b2 + c2 – 2bc cos A To minimize confusion, we'll rearrange the equation before plugging things in. 
Wonderful. Now we're ready to plug in our sides. 
Our calculator can handle the rest. A ≈ 51.3° Noice. Now we have an angle-side ratio, so we'll make use of the Law of Sines for the rest. 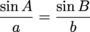
We just calculated A and we want to calculate B. 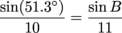
Rearrange the equation to solve for B. 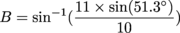
Calculate the angle. B ≈ 59.1° Sweet. Two down, one to go. A + B + C = 180°
51.3° + 59.1° + C = 180° For the last angle, we get: C = 69.6° That means the angles of the triangle are 51.3°, 59.1°, and 69.6°. |