For this one, we have to use our knowledge of both the magical right triangles. Gandalf and the Triforce unite, creating The Legend of the Lord Zelda's Ring. An Academy Award winner for sure. The only information we have is about the Gandalf triangle, so that's the best place to start. We know the formulas pretty well by now. 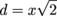
The hypotenuse, d, is  . . 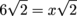
x = 6
We're off to a great start but where do we go from here? Both legs of the Gandalf triangle are equal to each other. Here's where Gandalf and the Triforce combine. The leg of the Gandalf triangle equals the length of the Triforce triangle's long leg. If we remember our Triforce triangle formulas, then it looks like this. 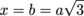
Since we just figured out that x = 6, that deserves some recognition. 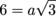
Now, we can solve for a, which will give us the length of the Triforce triangle's short side. 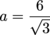
No one likes having square roots in the denominator. Luckily, we can fix that right up. 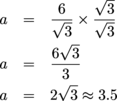
All that's left to do is to combine the sides of the triangles we need. Our value y is the sum of x (the leg of the Gandalf triangle), and a (the short leg of the Triforce triangle). y = a + x
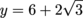
y ≈ 9.5 We might as well have a fork sticking out of us, because we're done. |