In order to find out whether these points make a right triangle or not, we need to find the distances between them. The distance between two points is given by the formula  , where d is the distance and x and y are the coordinates of points 1 and 2. We'll need to do this three times, so let's get to it. We'll start with the distance between A and B.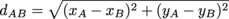 
Substituting in the coordinates, we get: 
Simplifying the numbers gives us: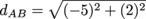 
Square the numbers and then add.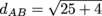 
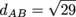
Since plugging this into our calculater will only give us an approximate, we'll leave the answer as-is for now. Next up, the distance from B to C: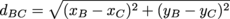 
Go through the same thing again, making sure to substitute the numbers in the right places.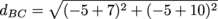 
Simplify, square, and add. 
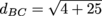
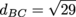
Hmmm. The same as dAB. We'll do this one last time for A and C.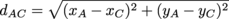 
Plug it in, plug it in. Then simplify, of course.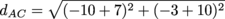 
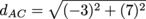
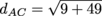
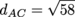
Whew. We figured out the distances between the points:  ,  , and  . Those are the lengths of the triangle's sides. They don't tell us much alone, but if these numbers satisfy the Pythagorean Theorem, that means the triangle is right. Wait. What's the Pythagorean Theorem, again? a2 + b2 = c2 Oh, yeah. Now plug in the distances. The hypotenuse, by default, is always the longest side so that will be c. And those square roots will get canceled out by the exponents. How much simpler can simplifying get? 29 + 29 ≟ 58 Wait for it. Wait for it… 58 = 58 Yes! That means ∆ABC is indeed a right triangle. Success has never smelled sweeter. | 
